Analysis Of Phasor Diagram Of Synchronous Motor
Consider a phasor diagram with normal excitation i.e. such a current through field winding which will produce flux that will adjust magnitude of Ebph same as Vph.
Let Ф be the load angle corresponding to the load on the motor. So from the exact opposing position of Ebph with respect to Vph. Ebph gets displaced by angle Ф.
Vector difference of Ebph and Vph, gives the phasor which represents Ia Zs, called ERph.
Now Zs = Ra + j Xs Ф
where Ra = Resistance of stator per phase
Xs = Synchronous reactance of stator per phase
i.e. Ф = tan-1 (Xs/Ra)
and | Zs| = Ф(Ra2 + Rs2) Ф
This angle ‘Ф’ is called internal machine angle or an impedance angle.
The significant of ‘Ф’ is that it tells us that phasor Iaph lags behind ERph i.e. Ia Zs by angle Ф. Current always lags in case of inductive impedance with respect to voltage drop across that impedance. So phasor Iaph can be shown lagging with respect to ERph by angle Ф. Practically Ra is very small compared to Xa and hence Ф tends to 90o.
Note : The power factor at which motor is running, gets decided by the angle between Vph and Iaphshown. This angle is denoted as Ф and called power factor angle.

and cos Ф = Power factor at which motor is working.
The nature of this p.f. is lagging if Iaph lags Vph by angle Ф. While it is leading if Iaph leads Vph by angle Ф. Phasor diagram indicating all the details is shown in the Fig.1.
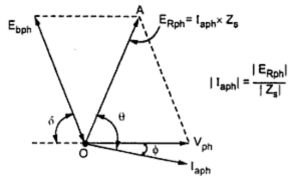 |
| Fig. 1 Phasor diagram under normal working condition |